Karcevskij conjecture 1928 and Kawamata conjecture 2002

TANAKA Akio

Sergej Karcevskij declared a conjecture for language's asymmetric structure on the TCLP of the Linguistic Circle of Prague in 1928. I briefly wrote about the conjecture as the following.

                          .....................................................................................................................................

Prague in 1920s, The Linguistic Circle of Prague and Sergej Karcevskij's paper "Du dualisme asymetrique du signe linguistique"

From Print 2012, Chapter 18

Non-symmetry. It was the very theme that I repeatedly talked on with C. Prague in 1920s. Karcevskij's paper "Du dualisme asymetrique du signe linguistique" that appeared in the magazine TCLP.  Absolutely contradicted coexistence between flexibility and solidity, which language keeps on maintaining, by which language continues existing as language.  Still now there will exist the everlasting dual contradiction in language. Why can language stay in such solid and such flexible condition like that. Karcevskij proposed the duality that is seemed to be almost absolute contradiction. Sergej Karcevskij's best of papers, for whom C called as the only genius in his last years' book Janua Linguisticae reserata 1994. 

Source: 

1. Tale / Print by LI Koh / 27 January 2012  

Reference:

1. Fortuitous Meeting, What CHINO Eiichi Taught Me in the Class of Linguistics / 5 December 2004 

Reference 2:

1. Linguistic Circle of Prague / 13 July 2012 - 19 July 2012

References 3:

1. Note for KARCEVSKIJ Sergej's "Du dualisme asymetrique du signe linguistique" / 8 September 2011
2. Condition of Meaning / 11 September 2011

References 4:

1. Dimension of Language / 4 September 2013
2. Synthesis of Meaning and Transition of Dimension / 6 September 2013

Reference 5:

1. Reversion Conjecture Revised / 1 May 2014 - 20 May 20

[Note, 2 October 2014]
In this Tale, Print 2012, C is CHINO Eiichi who was the very teacher in my life, taught me almost all the heritage of modern linguistics. I first met him in 1969 at university's his Russian class as a student knowing nothing on language study.

1. From Distance to Pseudo-Kobayashi Distance / 5 February 2012

Tokyo
23 February 2015
SIL
....................................................................................................................................

This asymmetric duality of linguistic sign presented by Karcevskij has become the prime mover for my study from the latter half of the 20th century being led by my teacher CHINO Eiichi.
But the theme was very hard even to find a clue. The turning point visited after I again learnt mathematics especially algebraic geometry in 1980s. 
In 2009 I successively wrote  the trial papers of the theme assisted by several results of contemporary mathematics. The papers are the following.

......................................................................................................................................

 

　　　　　.......................................................................................................................................................


Kontsevich's conjecture
Category theoretic mirror symmetry conjecture

When there exists mirror relation between X1 and X2, derived category of X1's  coherent sheaf  and derived Fukaya category defined from X2's symplectic structure become equivalence.

M. Kontsevich. Homological algebra of mirror symmetry, Vol. 1 of Proceedings of ICM. 1995.　　　　　　　　　　　　　　

　　　  ................................................................................................................................................


[Note by TANAKA Akio]
In the near future, symplectic geometry may be written by derived category. If so, complexed image of symplectic geometry's some theorems will become clearer.

References
Mirror Symmetry Conjecture on Rational Curve / Symplectic Language Theory / 27 February 2009Homological Mirror Symmetry Conjecture by KONTSEVICH
/ Symplectic Language Theory / 26 April 2009Quantization of Language / Floer Homology Language / 24 June 2009Tokyo
10 May 2016
SRFL Theory

　　　　　　...................................................................................................................................................

In the TODA's book, I received the great hint on Karcevskij's conjecture for language's hard problem.
The hint exists at Kawamata conjecture presented in 2002. The details are the following.

　　　　　　.....................................................................................................................................................

Derived Category Language 2
Kawamata Conjecture Conjecture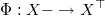is birational map between smooth objective algebraic manifolds.And.
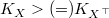At This condition,there exists next fully faithful embedding..

[Reference]

1. TODA Yukinobu. Several problems on derived category of coherent sheaf.  Tokyo, 2016.Chapter 6, Derived category of coherent sheaf and birational geometry, page 148, Conjecture 6.43.

[References 2]

1. Bridge across mathematics and physics
2. Kontsevich's conjecture Category theoretic mirror symmetry conjecture

[Reference 3]

1. Stability of Language / Language and Spacetime

 Tokyo19 May 2016SRFL Theory ......................................................................................................................................................................................... 

Kawamata conjecture will hint me the new meaning's entrance in the old meaning at a word. Notes for KARCEVSKIJ Sergej that I ever wrote will be newly revised through TODA's fine work over viewing the recent 20 year development on derived category that began by Grothendieck.  

For TODA's book, refer to the next my short essay.

...........................................................................................................................................

Bridge across mathematics and physics / Revised

 TODA Yukinobu. Several problems on derived category of coherent sheaf.  Tokyo, 2016 TODA Yukinobu's  Several problems on derived category of coherent sheaf has built across mathematics and physics. For my part, further more, physics and language seem to be expected to build over from the book.Chapter 5. Page 116. Conjecture 5.16 shows us the connection between symplectic geometry and algebraic geometry.I ever wrote several notes on language related with string theory.  Now TODA's book newly lights up the relation between physics and language. This relation is really fantastic for me from now on. [References]Distance Theory Algebraically Supplemented

1.      Bend     
2.      Distance 
3.      S3 and Hoph Map

 [References 2]Isomorphism of map Sequence / Symplectic Language Theory  Tokyo

19 May 2016

SRFL Theory .

Read more: https://srfl-theory.webnode.com/news/karcevskij-conjecture-1928-and-kawamata-conjecture-2002/